By now, you will already have a good understanding of the processes involved in planning and preparing to undertake a building and construction task or project. There is some specific information relevant to concreting that you will need for the planning and preparation stage.
Planning and preparing
The steps for planning and preparing are as follows:
- Reviewing plans and specs.
- Checking legislation, Standards, WHS and environmental requirements, workplace requirements.
- Check SWMS and SDS
- Selecting tools and equipment.
- Selecting PPE.
- Inspecting the worksite.
- Selecting materials and preparing the worksite
Reviewing plans and specs.
When reviewing the plans and specs, make sure you have thoroughly read and understood the plans and specifications and you know exactly where the concrete is required.
Checking legislation, Standards, WHS and environmental requirements, workplace requirements.
It is essential you have read and understood the relevant documentation prior to carrying out a task. If you are unsure or you have questions regarding the job and the compliance with legislation, standards, WHS and environmental requirements, you must address them and escalate it to your workplace supervisor.
Check SWMS and SDS
Ensure you are following the safety advice on the SWMS and SDS that has been stipulated for the task.
Selecting PPE
Ensure you have selected the appropriate PPE for the task you are conducting. The PPE needs to be in proper working order and must comply with the relevant Australian Standards for PPE. In this case you would require:
- safety hat
- a mask
- safety boots
- hi-vis clothing
- gloves
- possible eye protection.
Inspecting the worksite.
Before carrying out a task, you must make sure the site has had a thorough site inspection. This includes identifying any risks, no matter how small and erecting the appropriate signage or barricades where necessary.
Selecting tools and equipment.
Ensure you have access to the appropriate tools and equipment for the job. The tools and equipment must be thoroughly checked before you use them in case they are not in working order. If you find a piece of equipment that is not in working order, then you must tag it and remove it from use.
Selecting materials and preparing the worksite
After you have read through and understood the plans and specifications required for the task, you can determine what materials you will need to get yourself organised. When working with wet concrete, you must be relatively quickly, because of the setting process. You do not want the concrete to start to set because you are trying to organise the worksite as you go. It is also important you have thoroughly checked the quantities and materials you need; you do not want to under order or over order.
Identifying the materials that you will require
You will need to ensure that you have sufficient quantities of:
- timber for edge boards
- pegs for marking the areas
- cement to carry out the concreting work
- bar chairs
- bracing
- reinforcement mesh
- spacers
- vapour barriers have been appropriately sourced and comply with the NCC. The NCC stipulates for all Class 1 and Class 10 buildings, a vapour barrier is required under a slab-on-ground construction. 7
How much will you need?
The plans and specs for the job should contain much of the information that you need to calculate your requirements (for example, the perimeter of the area to be concreted, the depth of concrete etc).
Calculating formwork
When you are calculating formwork, there are some key concepts that you should keep in mind. These include:
- You will need to calculate the perimeter of the task.
- Timber for formwork can be expensive. So that you can reuse it, you should avoid cutting it where possible. You also need to allow for overlaps in the corners. This means that the amount of timber that you need for the job will exceed the perimeter.
- Remember that timber is available in increments of 300 mm (or 0.3 m).
- You should always account for waste in your calculations.
We will now take a look at the perimeter formulas you will need when calculating formwork.
Rectangle formula
When calculating the perimeter of a rectangle, you add the measurements of all sides of the rectangle, that is the length + width + length + width. Remember, a rectangle has two equal lengths and two equal widths, therefore the formula may appear as either:
- Perimeter (P) = Length (L)+ Width (W) + Length (L)+ Width (W)
- Perimeter (P) = 2 x (L + W)

Square formula
A square has four equal sides, so the measurements will be the same across all four sides, therefore you will only need to carry out the measurements for one of the sides of the square.
The formula for the perimeter of a square may appear as either:
- Perimeter (P) = Side 1 Length (L) + Side 2 Length (L) + Side 3 Length (L) + Side 4 Length (L)
- Perimeter (P) = 4 x Length (L)

Trapezium and triangle formula
When calculating the perimeter of a trapezium or a triangle, the method is the same. You will need the measurements of each side, then add them altogether.
A triangle:
Perimeter (P) = Side 1 + Side 2 + Side 3
A trapezium:
Perimeter (P) = Side 1 + Side 2 + Side 3 + Side 4
Formula of circles
The calculations of the circles are the more complex of all perimeter equations. Take the time to read through the process to ensure you understand.
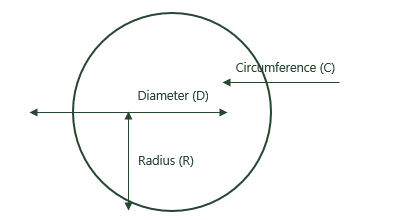
To calculate the perimeter of a circle (its circumference [C]), you need to know its diameter. The diameter of a circle is the measurement between its two widest points.
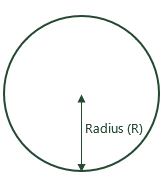
The radius of a circle is the measurement from its centre to its outside edge so you can calculate the diameter by doubling that number:
Formula for Diameter:
(D) = 2 x Radius (R)
To calculate the circumference of the circle, we use pi. Pi is the ratio of the circumference of a circle to its diameter. Regardless of the size of the circle, this ratio does not change. Pi is used as a decimal value in a number of mathematical calculations for circles and cylinders. You will see it written as pi or π.
Pi is an irrational number which means that its decimal form continues endlessly. So that we can use it easily, we round it off. Commonly to two or three decimal places. In this case, we have rounded it off to four decimal places which is 3.1416.
Formula for Circumference:
(C) = pi (π) x Diameter (D)
Calculating reinforcement
When you are calculating the amount of reinforcement that you will need, there are some key concepts that you should keep in mind. These include:
- You will need to calculate the area of the task.
- Each sheet of reinforcing mesh covers 1.8 m x 1.0 m (or 1.8 m2).
- You should allow for areas of overlap.
- Mesh reinforcement starts 50 mm in from each edge of the formwork, so you need to allow for this in your calculations.
We will now take a look at the formulas for calculating area.
Area is identified as the size of a surface. In building and construction, it is most commonly expressed in square metres (m2) or square millimetres (mm2). When you are thinking of area, consider the number of one metre x one metre (or millimetre) sheets of paper that you could fit on the surface that you are measuring.
Area formula for rectangles or squares
To calculate the area of a rectangular or square surface, you multiply length (L) by width (W). So, a sheet of cardboard that is 1 m x 1 m would have an area of 1 m2.
The formula appears as follows:
Area (A) = length (L) x width (w)
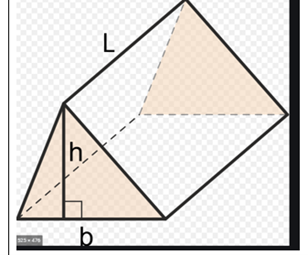
Area formula for triangles
When calculating the area of a triangle, you need to determine the measurements of the base and height. Once you have those calculations, you need to half the base and multiply it by the height.
The formula for calculating the area of a triangle may appear as either:
Area = ½ x (base x height)
Area = 0.5 x (B x H)
Area formula for a circle
When calculating the area of a circle, you need to determine the measurement of the radius; the radius being half the diameter.
The formula for the area of a circle may appears as either:
Area (A) = π x the square of the radius (R2). The square of the radius means the radius times itself. For example, if the radius was 300 mm, the square of the radius would be 300 mm x 300 mm.
So the formula is:
- Area (A) = π x R x R
- A = πR2
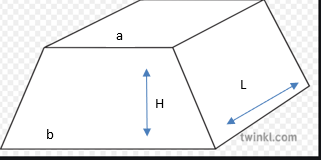
Area formula of trapezium
A trapezium (also known as a trapezoid) is a quadrilateral shape, meaning it has four sides, however, it is classified as irregular.
To calculate the area of a trapezium, you first need to add the measurements of a + b (the parallel sides) half it and multiply the answer to the measurement of the height.
The formula appears as either:
- A= 1/2 (a+b) x h
- A= 1/2 h (a+b)

Calculating volume
Volume is the amount of space within an object. This is particularly important to understand because you will use volume calculations to work out how much concrete you will need to fill the formwork for a slab. Volume is expressed in cubes. So cubic metres (m3) or cubic millimetres (mm3).
Formula for the volume of a cube
To calculate the volume of a cube (V), multiply the length (L) x width (W) x height (H).
The formula will appear as either:
Volume (V) = length (L) x width (W) x height (H)
V= L x W x H
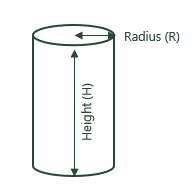
Formula of a cylinder
To calculate the volume of a cylinder, calculate the area of the circle at one end and multiply it by the height of the cylinder.
The formula will appear as follows:
V = (πR2) x H
Area of volume of trapeziums
When calculating the area of a trapezium you need to half the sum of parallel sides (a + b) then multiply the height (H) by the length (L).
The formula appears as follows:
V= ½ (a + b) x H x L
Area of triangular prisms
When calculating the area of a triangular prism, you need to work out the measurement of half of the base (b) and height (h), then multiply it by the length (l)
The formula appears as follows:
V = ½ x b x h x l